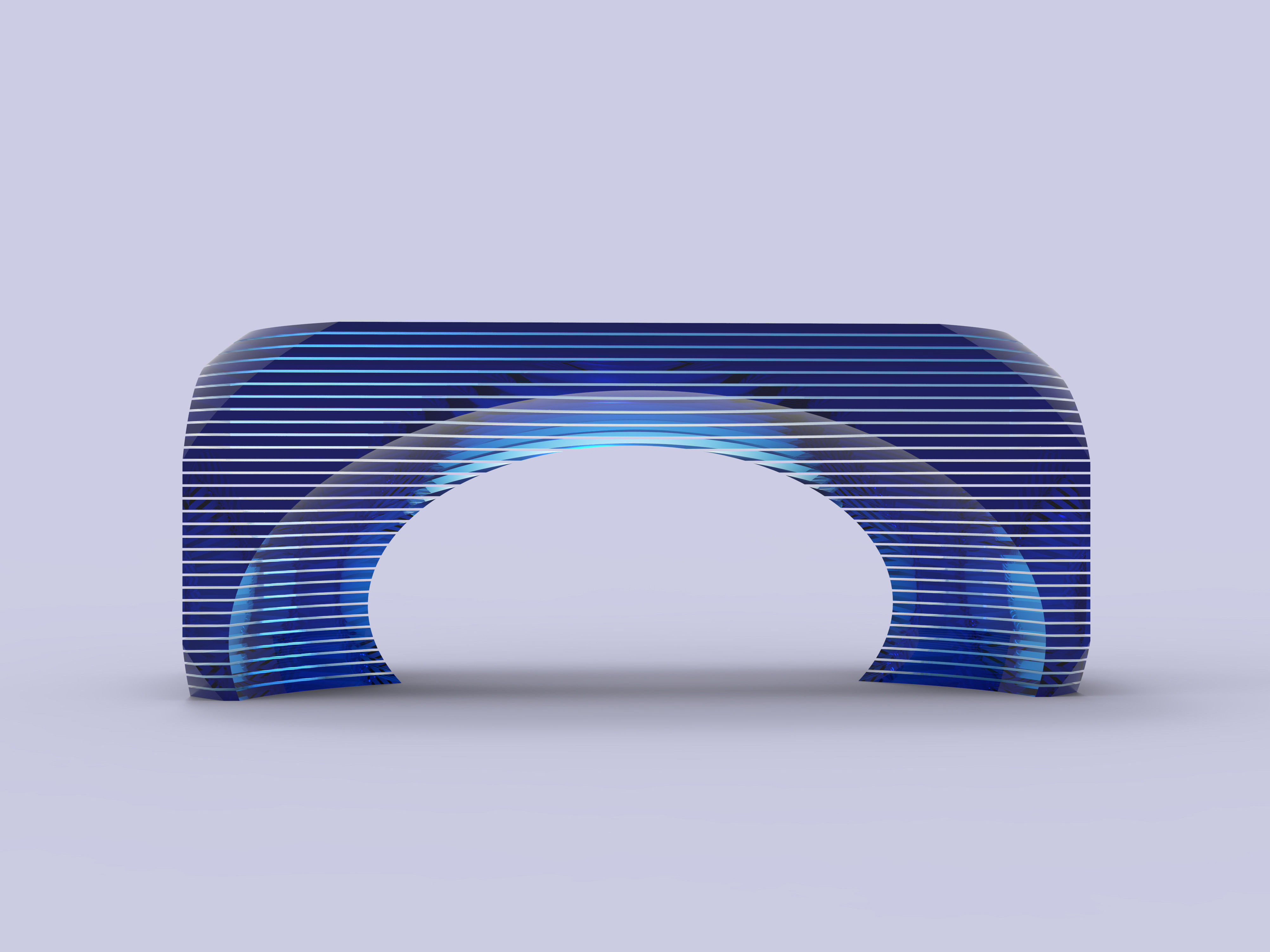
GOLDEN RECTANGLE

In geometry, a golden rectangle is a rectangle whose side lengths are in the golden ratio of 1:Φ (or "phi," approx 1.618). This ratio is found in some patterns in nature and is how many artists have composed their works. The origin of the ratio is unclear and some have found it dates back to the ancient Egyptians and the construction of their pyramids. During the 5th Century BC, Egyptian mathematician Euclid studied and obsessed over these proportions. It was ultimately popularized by Italian mathematician Fra Luca Bartolomeo de Pacioli in the 15th Century. Considered the Father of Accounting and Bookkeeping, he was a contemporary of Leonardo Da Vinci. This ratio is often referred to as the Fibonacci Sequence in nature.  These simple instructions create it: 1. Draw a square. 2. Draw a line from the midpoint of one side of the square to an opposite corner. 3. Use that line as the radius to draw an arc that defines the rectangle's height. 4. Complete the golden rectangle. This coffee table is made from welded and powder-coated steel with inset, smoked black glass. The shadow effect gives the table a minimalist appeal, but other color combinations will inspire different moods.